Building a Circulator out of Ceramics

---

Introduction

During my time as a PhD student, I had an opportunity to work in the laboratory of ceramic technologies. I got to produce various electronic components based on so-called low temperature co-fired ceramics, or LTCC. Although  it’s been more than 2 years since I left that lab, I found some time to reflect back on production of one of the most exotic components out there, a microwave circulator.

Crash course of circulator physics

A circulator is a pretty much irreplaceable component in microwave circuits, what automatically makes it a pretty expensive, too. When you push an EM wave at one of its three ports, it’s only going show up only at one of other two ports. And when you push the same wave on second port, it’s going to come out only at third, but not on the first. We say that the wave will circulate between the ports. This feature, fancy called non-reciprocity, appears thanks to a secret ingredient inside of it  – a ferrite. A ferrite is basically a ceramic (i.e. an insulator) which is, due to the presence of iron oxides, magnetically responsive. Ferrimagnetic, if you insist. It will attach to your magnet if you bring it close enough, but normally it will remain demagnetized. Like an iron needle, but non-conductive. Now the cool part: any transversal wave coming through the ferrite surface splits into two components: clockwise and counter-clockwise rotating components. This effect is known as the Faraday rotation, and to make it work we need one condition fulfilled: the ferrite disks to be magnetized by a static magnetic field vertically to the plane of rotation. But, the fun doesn’t end there. What makes ferrites non-reciprocal, that is, very useful in circulator design, is their anisotropy. Oh boy, another new word. Anisotropy of a material makes propagation in one direction through the material much easier than in the other. Result is that only one of two rotating components is really present there, and the incoming wave will really circulate around the applied field . The only thing left is to catch the circulating wave at certain port location and get it out of the whirlpool.

In case this section is not really hitting your math spot, check out this book for a more  mathematical description of circulator phenomena. I used it as a basis of my research back then. There’s a pretty decent intro to circulators in the microwave engineer’s all time favorite David Pozar’s book, too.

Design and simulation

Practical realizations for three-port circulators usually involve the symmetrical junction (Y-junction) of three identical waveguides or stripline TX lines, together with an axially magnetized ferrite disk placed at the center. A kind of circulator I was interested in consists of three striplines, placed 120° from one another, meeting at the center. The ferrite disks are placed above and below the circle, and everything is sandwiched between two conductive planes. This kind of circulator was firstly designed by a guy named Bosma in 1960s (paper excerpts below). For the first time in history, I was about to produce that kind of circulator in a thick film ceramic technology.

The original paper lies down the mathematical analysis of the fields within the circulator. Point of the field analysis is to get out a pretty looking mathematical expression of the electromagnetic fields at any point in the space. Usually, you start excitedly with the elegant Maxwell equations. Then, after three lines of imposing boundary conditions and n-th order Bessel functions you’re desperately looking for cheat tricks, simplifications and approximations. I, in an attempt to simplify mathematical content on this blog, will sum up the conclusions.  For the circulator in this case, where input ports are 120° apart, it is assumed that rotating field Hφ has constant magnitude over each coupling region and is zero along the remaining part of ferrite disk boundary.  Imposing condition that transversal E-field component Ez is 0 at φ = π we assure that there will be no coupling into the isolated port. This condition establishes a constraint on the ferrite disk radius R and coupling angle Ψ. The analysis of Bosma shows these parameters to be related as:

( is the wave number and K is rms value of susceptibility tensor elements. Yes, you read it well).

Many things have changed from 1960s. For one, we don’t need to rely solely on approximating mathematics, but we can do much more: computer simulation. We can draw a 3D model of our device, we can set up a physical rules and constraints and we can make computer calculate all we need to know about the device. This is why we do a finite element method (FEM) simulation before we go on and produce any piece of electronics. The goal of the simulation was to find geometry parameters like radius R, stripline widths W and Winit, and field H0 so that output is closest to the behaviour of ideal circulator as possible. This has been done by sweeping the parameters over the reasonably broad range of values and finding the local minima of S parameter function. The simulation was performed with the COMSOL Multiphysics software and its embedded RF module.

 

 

In the simulation output, we’ll see colorized distribution of EM fields within the drawn geometry. For instance, in the image above, there is an electrical field moving from one port to the second one. We also see that none of the field reaches the third port. That’s the magic of the FEM simulation as well as of the anisotropy. In the simulation post-processing, we can extract more useful data, such as S-parameters and optimize the geometry to meet our requrements.

Crash course on LTCC technology

Once we got our calculations and we have a clear (more or less) idea about what to build, it’s the time to go to the lab.  The circulator was fabricated in the Laboratory for ceramic technologies, and it consisted of LTCC tape preparation, laser cutting, screen stencil preparation using photolithographic methods, screen printing, lamination and, finally, co-firing.

LTCC sheet in unfired (sometimes called green) state is a thin flexible compound of ceramic or glass powder with organic composites. In order to allow the co-firing of metals with high electrical conductivity, the sintering temperature of the ceramic substrate has to be reduced well below the melting point of the individual metal (e.g. Cu: 1084°C, Ag: 962 °C, Au: 1064°C). Glass is added to the ceramic powder to fulfill the low temperature requirements while maintaining advantageous properties of high mechanical strength, high specific electrical resistivity and low dielectric loss. The most commonly glass used for LTCC substrates is borosilicate glass with the main constituents silica ( SiO2) and boron oxide (B2O3). Organic composites serve as the binders for maintaining the strength of the compound and increasing its formability; plasticizers that give the compound its plasticity and flexibility; dispersing agents that provide control of the pH of the compound; antifoaming agents to prevent the occurrence of foam; surface treatment coupling agents to improve poor wettability of ceramic powder by lowering its surface tension; and non-aqueous organic solvents. However, in the final firing process all organic composites are completely eliminated (burnt), leaving firm structure of sintered ceramic powder particles within all layers. Similar happens in the conductive paste: organic composites that are contained in the paste are burnt during the firing process leaving sintered particles of conductive material. It is obvious that, during the firing, the structure will experience certain shrinkage due to the loss of organic composites. Therefore, firing properties of both tape and conductive paste must be compatible in order to obtain the same shrinkage rate to prevent cracking of the conductive lines.

Green tapes are firstly laser cut with perforations and via holes. The via holes are later filled with the silver-based low viscosity paste with the help of semiautomatic pneumatic stencil printer with the squeegee. Subsequently, conductive lines are screen-printed on each tape separately using the same printer. For adequate line printing, 30 µm thick Murakami stencil is attached on a steel screen with mesh of 450 dpi. The stencil was made with standard photolithography technique. Screen printing was performed with Heraeus TC7306 silverbased paste under pressure of 2.7 bars. After printing, tapes were dried at a temperature of 75 ◦C. Resulting line thickness after firing is typically 10 to 15 µm Printed tapes were stacked together and properly positioning with the help of the four additional reference holes which were fixed to four reference pins fastened on a metal mount. Once stacked and preheated to 80◦C, the structure was exposed to a uniaxial pressure of 80 – 160 bars depending on the structure’s number of layers for 3.5 minutes. During this process, called lamination, single substrate is created from stacked layers of material.

Co-firing of the laminated structure is the most sensitive process in LTCC production cycle. During the co-firing, ceramic and conductor particles are sintered together by exposition to the high temperatures. The most important technical points in this process are controlling the firing shrinkage of the whole substrate, controlling firing behavior of different materials within the sample to prevent defects, and achieving both, antioxidation of the conductor material (silver) and melting of the binder material (glass granules). Because of variety in sizes of samples, one must adjust firing parameters for each sample to satisfy these requirements.

(Perils of) Building a circulator

The tapes were prepared according to the process described in the previous section with the special attention taken for the mismatch of the shrinkage between two tapes. Layout of cut green tapes is given in the figures below.

A task to find optimal lamination and firing parameters was the most challenging part of this work. Many trials were taken in co-firing dielectric substrate and ferrite disk, but  results were disappointing. The significant mismatch in thermo-mechanical properties of two different tapes caused the breakage and deformation of the sample every time the co-firing was performed. Presence of silver paste, with its own sintering properties, caused ever worse results.

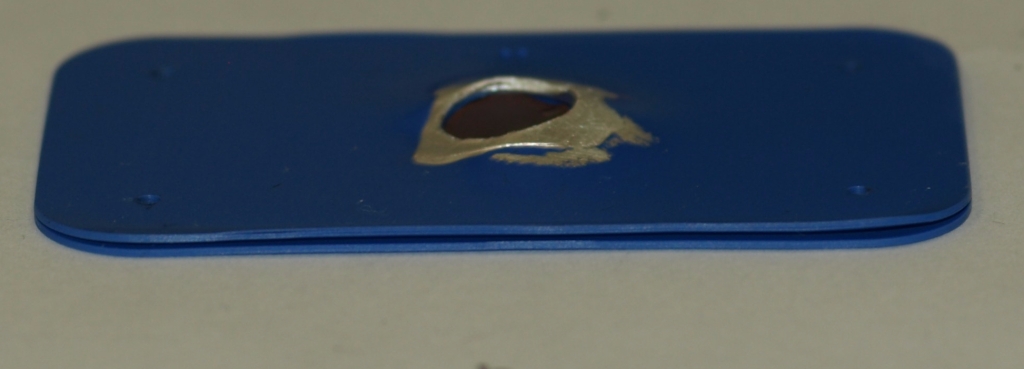
Behavior of the LTCC tapes and silver is best shown on the microscopic photo of the cross-section, where it is visible how the dielectric bends around the ferrite. This photo shows that ferrite sinters into its final form sooner than dielectric, forcing the dielectric to mold around it. Also, detachment of silver track of the ferrite is clearly visible.

Final approach in fabricating the circulator was independent pre-sintering of ferrite tapes followed by joint firing with dielectric tapes. Firstly, the disk-shaped ferrite tapes were laminated and fired in box furnace at 885◦C for 3 hours. The fired ferrite disks were placed in the dielectric substrate and fired again, but on the temperature profile suited for dielectric tapes. This way breaking of sample was prevented, although small deformations were still observable in the disk area. These were slightly reduced by additional supporting dielectric tapes on top and bottom of the sample. The supporting tapes also prevent deformation caused by the large silver area of the ground planes.

 

Measurements and characterization

Building the circulator was all fun and joy, but now we have to see if it’s worth anything.  Panel SMA connectors were attached to the ends of the circulator’s striplines and small disk-shaped neodymium magnets were placed on top and bottom of the ferrite. Measurements and characterization were performed on vector network analyzer Agilent E8364A in range of 4.5 – 8 GHz.

 

The following figures present dB scale graphs of transmission S-parameters (Sxy) and reflection S-parameters (Sxx). We see that for a certain frequency bad (4.7 – 5 GHz) there is clear circulation behavior. In that band, reflection is low at all ports and transmission is close to 0 dB only for waves traveling from port 2 to port 1, from port 1 to port 3 and from port 3 to port 2. For waves traveling from 1 to 2, 3 to 1 and  2 to 3, transmission is less than -30 dB. This is some nice circulation, isn’t it?